The Comonad.Reader

Grant B. asked me to post the derivation for the right and left Kan extension formula used in previous Kan Extension posts (1,2). For that we can turn to the definition of Kan extensions in terms of ends, but first we need to take a couple of steps back to find a way to represent (co)ends in Haskell.

(more...)

I want to spend some more time talking about Kan extensions, composition of Kan extensions, and the relationship between a monad and the monad generated by a monad.

But first, I want to take a moment to recall adjunctions and show how they relate to some standard (co)monads, before tying them back to Kan extensions.

Adjunctions 101

An adjunction between categories and consists of a pair of functors 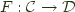, and and a natural isomorphism:

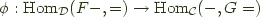
We call 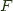the left adjoint functor, and 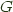the right adjoint functor and 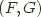an adjoint pair, and write this relationship as

(more...)

I think I may spend a post or two talking about Kan extensions.

They appear to be black magic to Haskell programmers, but as Saunders Mac Lane said in Categories for the Working Mathematician:

All concepts are Kan extensions.

So what is a Kan extension? They come in two forms: right- and left- Kan extensions.

First I'll talk about right Kan extensions, since Haskell programmers have a better intuition for them.

(more...)

 
> import Control.Arrow ((|||),(&&&),left)
> newtype Mu f = InF { outF :: f (Mu f) }
 

I want to talk about a novel recursion scheme that hasn't received a lot of attention from the Haskell community and its even more obscure dual -- which is necessarily more obscure because I believe this is the first time anyone has talked about it.

Jiri Adámek, Stefan Milius and Jiri Velebil have done a lot of work on Elgot algebras. Here I'd like to translate them into Haskell, dualize them, observe that the dual can encode primitive recursion, and provide some observations.

You can kind of think an Elgot algebra as a hylomorphism that cheats.

 
> elgot :: Functor f => (f b -> b) -> (a -> Either b (f a)) -> a -> b
> elgot phi psi = h where h = (id ||| phi . fmap h) . psi
 

(more...)

Does anyone know of any work on "forgetful laziness?"

(more...)

Ok, I decided to take a step back from my flawed approach in the last post and play with the idea of power series of functors from a different perspective.

I dusted off my copy of Herbert Wilf's generatingfunctionology and switched goals to try to see some well known recursive functors or species as formal power series. It appears that we can pick a few things out about the generating functions of polynomial functors.

As an example:

 
Maybe x = 1 + x
 

Ok. We're done. Thank you very much. I'll be here all week. Try the veal...

For a more serious example, the formal power series for the list [x] is just a geometric series:

(more...)

The post below will only compile on a version of GHC >= 6.9, since it uses type families.

(more...)

I did some digging and found the universal operations mentioned in the last couple of posts: unzip, unbizip and counzip were referenced as abide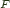, abide 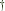and coabide 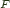-- actually, I was looking for something else, and this fell into my lap.

They were apparently named for a notion defined by Richard Bird back in:

R.S. Bird. Lecture notes on constructive functional programming. In M. Broy, editor, Constructive Methods in Computing Science. International Summer School directed by F.L. Bauer [et al.], Springer Verlag, 1989. NATO Advanced Science Institute Series (Series F: Computer and System Sciences Vol. 55).

The notion can be summed up by defining that two binary operations and abide if for all a, b, c, d:

.

There is a cute pictorial explanation of this idea in Maarten Fokkinga's remarkably readable Ph.D dissertation on p. 20.

The idea appears again on p.88 as part of the famous 'banana split' theorem, and then later on p90 the above names above are given along with the laws:

 
fmap f &&& fmap g = unfzip . fmap (f &&& g)
bimap f g &&& bimap h j = unbizip . bimap (f &&& h) (g &&& j)
fmap f ||| fmap g = fmap (f ||| g) . counfzip
 

That said the cases when the inverse operations exist do not appear to be mentioned in these sources.

Twan van Laarhoven pointed out that fzip from the other day is a close cousin of applicative chosen to be an inverse of the universal construction 'unfzip'.

During that post I also put off talking about the dual of zipping, so I figured I'd bring up the notion of choosing a notion of 'cozipping' by defining it as an inverse to a universally definable notion of 'counzipping'.

(more...)

Kefer asked a question in the comments of my post about (co)monadic (co)strength about Uustalu and Vene's ComonadZip class from p157 of The Essence of Dataflow Programming. The class in question is:

 
class Comonad w => ComonadZip w where
     czip :: f a -> f b -> f (a, b)
 

In response I added Control.Functor.Zip [Source] to my nascent rebundled version of category-extras, which was posted up to hackage earlier today.

(more...)